The Effect of bcc lattices on the Drug Release Kinetics in Inert Systems by Monte Carlo Simulation

Abstract

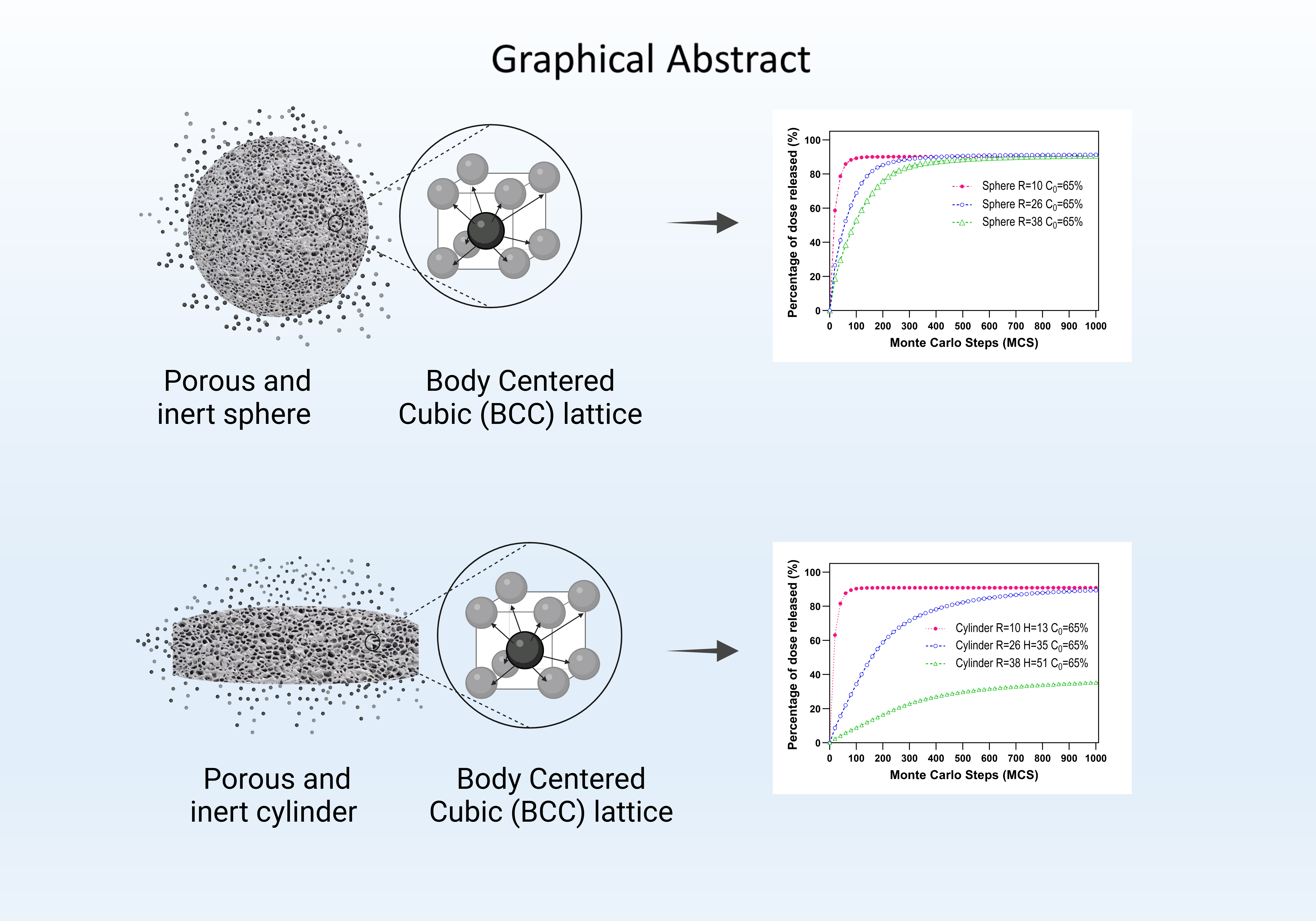
This study examines the release kinetics of hydrophilic drugs from inert and porous matrices structured as body-centered cubic (bcc) lattices, utilizing Monte Carlo simulations for analysis. In this research, we examined a sphere with three distinct radii and a cylinder with three varying height-to-radius ratios. For each sample, we assessed the kinetics of drug release at varying drug concentrations and modeled the release by simulating the random diffusion of drug particles to the device's boundaries. The comparison of release profiles highlighted the influence of size, geometry, and connectivity on the kinetic parameters and essential properties. Enhancing the area-to-volume ratio leads to a diminished rate of drug release. Similarly, an escalation in size, as indicated by the ratio 1:18:55, results in a reduced drug release rate. Additionally, our findings reveal that the quantity of drug retained indefinitely is greater within a body-centered cubic (bcc) lattice matrix compared to a simple cubic (cs) lattice structure. In both geometrical configurations, the trapped drug is independent of the system's scaling in comparison to a cs lattice. Furthermore, our analysis reveals that at larger scales, with a drug concentration above the theoretical percolation threshold, our system remains stable. The outcomes align with the empirical Higuchi equation and the Weibull function. Our findings concur with previously published experimental outcomes, suggesting that bcc connectivity is a reliable parameter for simulating diffusion processes in the drug release from solid pharmaceutical forms. This correlation supports the use of bcc connectivity as a predictive tool in pharmaceutical research, aiding in the understanding of drug release mechanisms.

Resumen. Este trabajo analiza la cinética de liberación de fármacos hidrófilos a partir de matrices inertes y porosas en una red cúbica centrada en el cuerpo (bcc) mediante simulacion de Monte Carlo. Para este estudio, seleccionamos una esfera con tres radios diferentes y un cilindro con tres relaciones altura/radio diferentes. Para cada uno, determinamos la cinética de liberación del fármaco con diferentes cargas y simulamos la liberación a través del movimiento aleatorio de cada partícula del fármaco hacia los límites del dispositivo mediante un proceso de difusión. Se compararon los perfiles de liberación y analizamos el efecto de escalamiento, la geometría y la conectividad sobre los parámetros cinéticos y las propiedades críticas del sistema. Al aumentar la relación área/volumen, disminuye la tasa de liberación del fármaco, mientras que con el aumento del tamaño (1:18:55), la tasa de liberación del fármaco disminuye. Además, identificamos que la cantidad de fármaco atrapado a tiempo infinito es mayor en la matriz constituida por la red bcc que en la red cúbica simple (cs). En ambas geometrías, bajo una red bcc se observó que la cantidad de fármaco atrapado no es sensible al escalamiento del sistema en comparación con una red cs. Además, caracterizamos nuestros sistemas mostrando que en escalas mayores y con una carga de fármaco muy por arriba del umbral de percolación teórico, los datos se ajustan a la ecuación empírica de Higuchi y la función de Weibull. Nuestros datos concuerdan resultados experimentales y teóricos previamente reportados, lo que permite considerar la conectividad bcc como un buen parámetro de simulación de procesos difusivos, como la liberación de fármaco desde formas farmacéuticas sólidas.