Non-Relativistic Ro-Vibrational Energies of Chlorine Molecule for Molecular Attractive Potential Model
DOI:
https://doi.org/10.29356/jmcs.v66i2.1712Keywords:
Wave equation, eigensolutions, bound state, Schrὅdinger equationAbstract
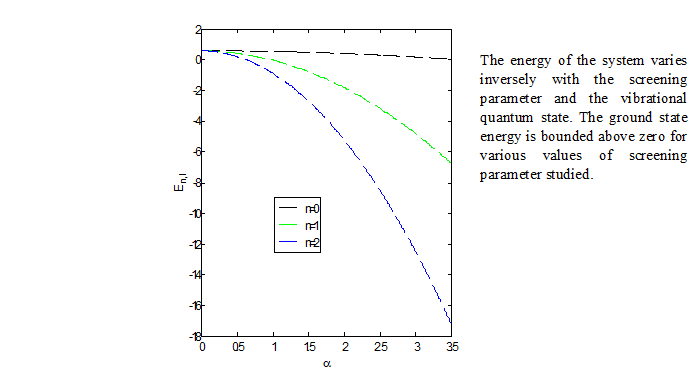
Abstract. We obtained the solutions of the radial Schrödinger equation with the modified molecular attractive potential model by employing the supersymmetric WKB method, and present the non-relativistic rotation-vibrational energy equation for diatomic molecules. Using the energy equation and the spectroscopic parameters of chlorine molecule, we computed the vibrational energy eigenvalues for various quantum states. The calculated results are found to be in agreement with the experimental values.
Resumen. Obtenemos las soluciones de la ecuación radial de Schrödinger con el modelo de potencial molecular atractivo modificado empleando el método WKB supersimétrico, y presentamos la ecuación para la energía rotacional-vibracional norelativista para moléculas diatómicas. Utilizando la ecuación para la energía y los parámetros espectroscópicos de la molécula de cloro, calculamos los valores propios de las energías vibracionales para varios estados cuánticos. Se encuentra que los resultados calculados están en acuerdo con los valores experimentales.
Downloads
References
Jia, C. –S.; Cao, S. –Y. Bull. Korean Chem. Soc. 2013, 34, 3425-3428. DOI: http://dx.doi.org/10.5012/bkcs.2013.34.11.3425 DOI: https://doi.org/10.5012/bkcs.2013.34.11.3425
Yanar, H.; Aydogdu, O.; Saltı M. Mol. Phys. 2016, 114, 3134-3142. DOI: http://dx.doi.org/10.1080/00268976.2016.1220645 DOI: https://doi.org/10.1080/00268976.2016.1220645
Bin Tang, B.; Jia, C. –S.. Eur. Phys. J. Plus. 2017, 132, 375. DOI: http://doi.org/10.1140/epjp/i2017-11657-7 DOI: https://doi.org/10.1140/epjp/i2017-11657-7
Khordad, R.; Ghanbari, A. J. Low Temp. Phys. 2020, 199, 1198. DOI: https://doi.org/10.1007/s10909-020-02368-8 DOI: https://doi.org/10.1007/s10909-020-02368-8
Liu, J. –Y.; Hu, X. –T.; Jia, C. –S. Can. J. Chem. 2014, 92, 40. DOI: dx.doi.org/10.1139/cjc-2013-0396 DOI: https://doi.org/10.1139/cjc-2013-0396
Onate, C. A.; Akanbi, T. A.; Okon, I. B. Scientific Reports. 2021, 11, 6198. DOI: https://doi.org/10.1038/s41598-021-85761-x DOI: https://doi.org/10.1038/s41598-021-85761-x
Onate, C. A.; Akanbi, T. A. Result. Phys. 2021, 22, 103961. DOI: https://doi.org/10.1016/j.rinp.2021.103961 DOI: https://doi.org/10.1016/j.rinp.2021.103961
Zhang, L. –H.; LI, X. –P.; Jia, C. –S. Int. J. Quant. Chem. 2011, 111, 1870. DOI: http://dio.org/10.1002/qua.22477 DOI: https://doi.org/10.1002/qua.22477
Qiang, W.-C.; Dong, S.-H. Physics Letters A. 2007, 368, 13. DOI: doi:10.1016/j.physleta.2007.03.057 DOI: https://doi.org/10.1016/j.physleta.2007.03.057
Dong, S.-H; Qiang, W.-C. Phys. Lett. A. 2008, 372, 4789. DOI: doi.org/10.1016/j.physleta.2008.05.020
Greene, R. L.; Aldrich, C. Phys. Rev. A. 1976, 14, 2363-2366. DOI: https://doi.org/10.1103/PhysRevA.14.2363 DOI: https://doi.org/10.1103/PhysRevA.14.2363
Jia, C. –S.; Guo, P.; Diao, Y. –F.; Yi, L. –Z.; Xie, X. –J. Eur. Phys. J. A. 2007, 34, 41-48. DOI: http://doi.org/10.1140/epja/i2007-10486-2 DOI: https://doi.org/10.1140/epja/i2007-10486-2
Hassanabadi, H.; Zarrinmakar, S.; Rahimov, H. Commun. Theor. Phys. 2011, 56, 423. DOI: http://www.iop.org/EJ/journal/ctp DOI: https://doi.org/10.1088/0253-6102/56/3/05
Wei, G. –F.; Dong, S. –H. Phys. Lett. B. 2010, 686, 288. DOI: http://doi.org/10.1016/physletb.2010.02.070
Witten, E. Nucl. Phys. B. 1981, 188, 513. DOI: https://doi.org/10.1016/0550-3213(81)90006-7 DOI: https://doi.org/10.1016/0550-3213(81)90006-7
Gendenshtein, L. E. Sov. Phys. (JETP) Lett. 1983, 38, 356.
Cooper, F.; Khare, A.; Sukhatme, U. Phys. Rep. 1995, 251, 267. DOI: https://doi.org/10.1016/0370-1573(94)00080-M DOI: https://doi.org/10.1016/0370-1573(94)00080-M
Comtet, A.; Bandrank, A.; Campbell, D. E. Phys. Lett. B. 1985, 150, 159. DOI: https://doi.org/10.1016/0370-2693(85)90160-1 DOI: https://doi.org/10.1016/0370-2693(85)90160-1
Coxon, J. A. J. Quant. Spectrosc. Radial Transfer. 1971, 11, 443-462. DOI: https://doi.org/10.1016/0022-4073(71)90083-5 DOI: https://doi.org/10.1016/0022-4073(71)90083-5
Kaur, S.; Mahajan, C.G. Pramana J. Phys. 1999, 52, 459. DOI: https://doi.org/10.1007/BF02830093 DOI: https://doi.org/10.1007/BF02830093
Downloads
Published
Issue
Section
License
Copyright (c) 2022 C. A. Onate, I. B Okon, M. C Onyeaju
This work is licensed under a Creative Commons Attribution-NonCommercial 4.0 International License.
Authors who publish with this journal agree to the following terms:
- Authors retain copyright and grant the journal right of first publication with the work simultaneously licensed under a Creative Commons Attribution License that allows others to share the work with an acknowledgement of the work's authorship and initial publication in this journal.
- Authors are able to enter into separate, additional contractual arrangements for the non-exclusive distribution of the journal's published version of the work (e.g., post it to an institutional repository or publish it in a book), with an acknowledgement of its initial publication in this journal.
